In the plane of the CCD, the spectrum scale, expressed in A/mm (Angstroms by millimeter) and also know as plate factor P, can be computed as
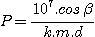
with d the distance between the grating and the focal plane. If we assume that d=21 mm and that we use the first order (k=1) with m=100 grooves/mm, then we have b=3,7░ for a wavelength of 0,65 micron and the plate factor is P=4752 A/mm. It can be verified that the spectrum scale is practically independent of the wavelength, which is nice for data processing.
The CCD being sensitive between 4000 A and 9000 A, the linear extension of the spectrum is (9000-4000)/4752=1,05 mm. If the CCD is a KAF-0400, with pixels 9 microns on a side, computing the spectrum length in pixels is easy: 1,05/0,009=117 pixels. In general if e is the linear size of a pixel, then this pixel covers a spectral element whose size is:
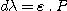
In our example, dl=9.10-3 . 4752 = 43 A.
One should not distinguish between the value of spectrum sampling, as previously computed, with the spectral resolution. The theoretical value of the latter is given by:
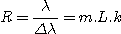
with
m = the number
of grooves per millimeter
L = the grating's width (along a direction perpendicular
to grooves direction)
k = the grating's order
With d the distance between the focal plane and the grating, the grating's width when observing a pointlike object is:
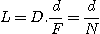
where D is the telescope's diameter, F its focal length and N is the F/D aperture ratio. If D=190 mm, F=760 mm (N=4) and d=21 mm, then we find L=5,25 mm and a resolution of 525. This must be understood as a theoretical resolution, since in practice the grating comes with aberrations that will lower it.
The main aberration affecting our system is chromatic coma. As its name implies it depends on wavelength. The dimension of the coma as a function of the wavelength is given by
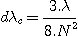
or, expressed as a linear dimension in the plane of the spectrum:
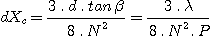
With chromatic coma, resolution is expressed by
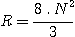
We note that resolution is independant of the grating's grooves number and of the grating to focus distance.
With N=4 and l=6500 A, the chromatic coma is 152 A, equivalent to an aigrette's length of 0,032 mm. Resolution is then 43. The only way to improve things is to use a slower telescope, but this is less than ideal. First because reducing the field's size is not a good idea if it is desired to observe many objects at the same time, and also because increasing the focal length also increases star sizes, which in turn implies a loss in resolution (read further on).
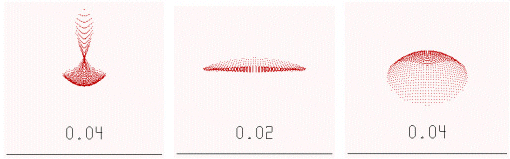
Figure below shows image spots in the focal plane for 3 wavelengths, computed by ray-tracing in the example's optical configuration.

Spot-diagrams
computed by ray tracing on a system comprising a grating with 100
grooves/mm, placed 21 mm from the focal plane in a converging beam
@ F/D=4. From left to right, wavelength is 0,45 micron, 0,65
micron, and 0,80 micron. The horizontal dash is equivalent to 0,1
mm. The dispersion axis is vertical, the spectral lines being horizontal.
A typical characteristic of a coma, as is visible in the upper figure, is that 80% of the energy is in fact concentrated in one half of the image spot. Hence the previous formulae give a pessimistic result. They are often replaced by the following formulae that better take into account the energy repartition in the image spot:
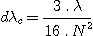
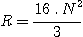
With these new assumptions, the chromatic coma is only 76 A and the resolution reaches 86.
Images on the upper figure are spoiled by some astigmatism. In our disposition it has a linear dimension of
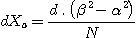
where a and b are expresssed in radians.
If a = -b, astigmatism is null for the wavelength l0 corresponding to emergence angle b. To this end one must give the grating an angle such that incidence and diffraction angles be equal, as is shown in this figure:
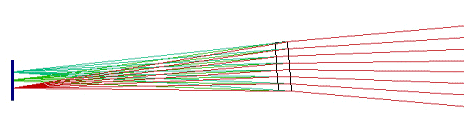
Grating
angle that corrects for astigmatism. Note how the angle is oriented
with respect to the diffraction angle for the order in use.
The a angle that corrects for astigmatism at wavelength l0 is given in radian by
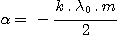
In a spectrograph astigmatism only lowers spectral resolution by a small amount if the detector's surface is located in the same plane as the tangential focal. However, widening in a direction perpendicular to dispersion may cause a loss in detectivity. In our example, widening of the spectrum at l=0,65 micron induced by astigmatism is 0,024 mm, of the same order of magnitude as chromatic coma. Hence giving an angle to the grating with respect to the optical axis gives little benefit with such assumptions.

The
image spot at 0,65 microns with a grating angle of about 2░
with respect to the optical axis.
The last aberration affecting our system is field curvature, due to the fact that the spectrum does not have a planar shape but rather a cylindrical one. Of course, since the CCD detector is flat, focus cannot be readily achieved for every wavelength in the spectrum. Because of astigmatism, there are in fact two focal surfaces, one related to the sagittal image (the image of a pointlike object is lengthened along the dispersion direction), the other related to the tangential focal (the image is lengthened perpendicularly to the dispersion direction), with a curvature radius of d/3. It is this last surface that we wish to position as close to possible of the CCD plane by focusing the telescope, since the spectral lines are themselves perpendicular to the dispersion direction.
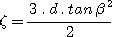
At the wavelength of 0,65 micron (b=3,7░) and for d=21 mm one finds 0,13 mm, which is not to be neglected. Hence, one should not insist on focusing the order 0 image with such a spectrograph, unless the CCD camera is given a slight angle with respect the optical axis so the detector's plane intercepts at the same time the best focus position for order 0 and a given point of the spectrum.
It is possible to greatly improve the quality of our spectrograph by adding an optical component: a small angle prism with one side positioned against the grating. If the latter is directly engraved on one face of the prism, then we have an optical element called a GRISM, a contraction of the words "grating" and "prism". The presence of the prism completely cancels chromatic coma for a chosen wavelength l0.
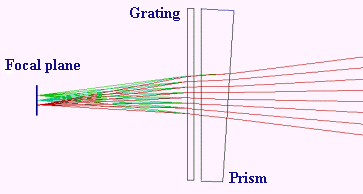
A prism is located close to the grating in order
to suppress coma. The deviation angle of the optical association
is practically null.
If n is the refraction index for the prism, it can be shown that the prism's angle g is expressed by
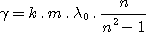
With our system and for n=1,5 one finds g =4,3░.
The length of the residual coma's aigrette for another spectrum point at wavelength l is
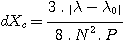
The spectrograph's resolution is then in the order of 30A on the full spectrum (see following figure).
Spot-diagram
computed by ray tracing through a system comprising a grating at
100 grooves/mm, placed 21 mm from the focal plane and a prism with
an angle of 3,87░, for a beam converging at F/D=4. From left
to right, wavelength is 0.45 micron, 0.65 micron and 0.8 micron.
Coma is nearly absent at 0.65 micron and only a fairly pure astigmatism
remains. The dimensions of the image spots are such that it is the
pixel size (or the seeing) that limits the resolution to a value
of about 25 A. The prism was carefully selected: it can be found
in many optical components catalogs, such as that from Melles Griot with reference 02PRW003 (1390 F
with taxes), or from Edmund Scientific (cheaper, about 35$).
Finally, it is very important to remember that the FWHM (Full Width at Half Maximum) directly determines spectral resolution in a slitless spectrograph. Tracking quality shall be maximized, also the telescope should be properly focused and tuned for optical quality. Turbulence itself should be low. For all these reasons, it is often easier to obtain good spectra with short focal lengths than with longer ones (a simple zoom lens corrected for chromatism can be as efficient as a 200 mm telescope for spectral resolution). If the figure for FWHM is 2 pixels and dispersion is 47A/pixel, then it will not be possible to reach a better spectral resolution than 2 x 47 or 94 A.
Using numerical applications as described in this page, taking into account the dimensions of your CCD detector, with the goal of studying weak objects' spectra, you should find that the best grating to be used will have at most 70 to 200 grooves per millimeter!
3. AN OPTIMAL GRISM ARRANGEMENT
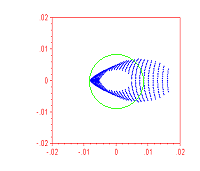
Definitively, when placing the grating very close to the CCD, image quality feels. Figure below shows the spots diagrams for several wavelength when there engraved surface of the Rainbow grating is placed 8 mm in front of the surface of the CCD (dispersion of 55 A/pixel).
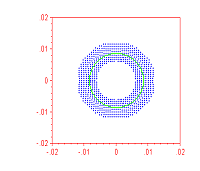
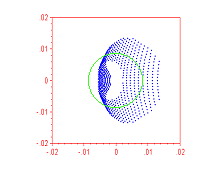
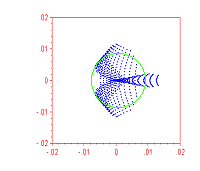
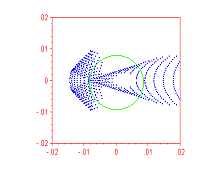
Spot-diagrams calculated in a configuration
where the the Rainbow Optics grating is located 8 mm in front of
the CCD. Telescope caracteristics: focal length of 760 millimeters,
F/D=4, and central obstruction of 0,4. From up left to dow right,
zero order image, 300 nm image, 500 nm image, and 700 nm image.
The green circle simulates the size of a star (FWHM) covering two
pixels when one uses a KAF-0400 CCD (9-microns pixels size). This
equivalent to a seeing of about 4 arc-seconds. Graduations are in
millimeters.
The analysis of this images shows a chromatic coma which one can reduce by introducing the wedge prism of small angle into the beam, just in front of grating. This prism can be integrated easily in the barrel of the Rainbow grating if it has a diameter of 25 mm.

Top, the Rainbow Optics transmission grating. Bottom, a 3░52' wedge prism (origin Edmund Scientific).

The 25 millimeters diameter prism into the Rainbow barrel (grism configuration).Note that the orientation of the prism relative to the grating grooves is critical. Nominally, the deviation of the prism compensate the deviation of the grating at the central wavelength
With a prism of angle 3.87░ (H43651 reference
in the Edmund Scientific catalog, material: BK7, thickness: 3.0
mm) one obtains the spots next images.
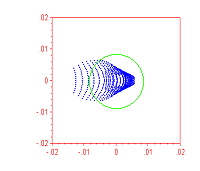
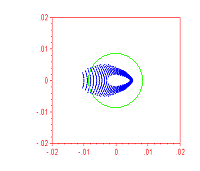
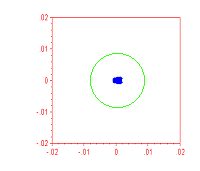
Same convention that next spot-diagrams.
The use of a prism in front of the Rainbow grating reduce the chromatic
coma appreciably. The field curvature is now the prevalent aberration.
The CCD was focused for the wavelength of 0,5 Ám where the
image is quasi stigmatic. The residual coma can be cancelled by
choosing a prism of more large angle (about 5 or 6░), but under
these conditions the astigmatism increases quickly and the benefit
of the correction of the chromatic coma cancels. The compromise
carried out here is almost ideal. The aberrations are now
of the same order of the seeing. The theoritical spectral resolution
is of 9 nm.
Goto index page Previous page Next page